This World War II aerial gunner training film distilled the mathematics of a deflection shot into an informative and entertaining cartoon. While the film was concise by nature, this piece endeavours to provide some background information for the modern audience.
Bore Sight Distance
A cursory search did not yield documentations detailing the B-17 50-calibre bore sight distances. However, WWII era USAAF manual 200-1* recommended a 300 to 400 yard harmonization range for fighter aircraft armament. The procedure converged the point of impact at that distance by correcting the parallax between the pilot and wing mounts, and compensating for bullet drop from disparate armament (e.g. 50-calibre and 20mm of the P-38, or the different barrel lengths on a P-47). The pilot then used this convergence zone as the optimal engagement distance. It is reasonable to assume similar ranges for B-17 armament as well.
Using a 400 yard bore sight distance, a 50-calibre projectile rises less than 1ft and drops less than 3ft inside of 600 yards . Since this deviation is smaller than the head-on profile of most fighters, elevation compensation becomes a negligible factor. For reference, the BMW 801 engine of a FW 190 has a 4.25ft diameter.
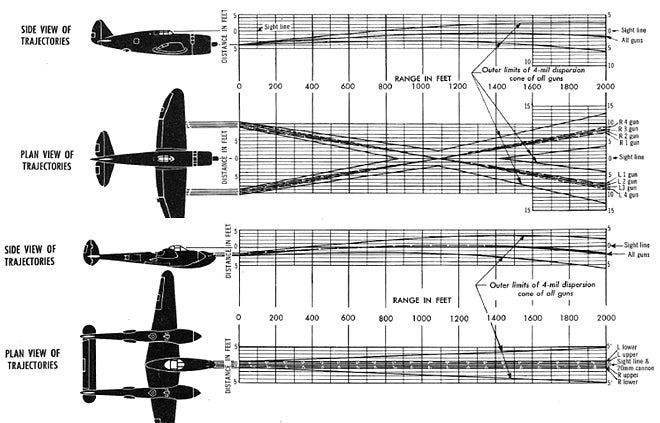
Convergence zones for P-47 (top) and P-38 (bottom)
Rule of “3, 2, 1”
The film provided gunners with the rule of three radii lead at 90o deflection, two radii at 45o, one radius at 22.5o, and half radius at 11.25o. This is derived from the fact that relative crossing velocity is proportional to the sine of the line-of-sight angle (λ). The following subsections step through the mathematics using an Earth Centered, Earth Fixed coordinate frame in two dimensions. The closing velocity vector is derived and the resulting trigonometric relationship examined.
Closing Velocity
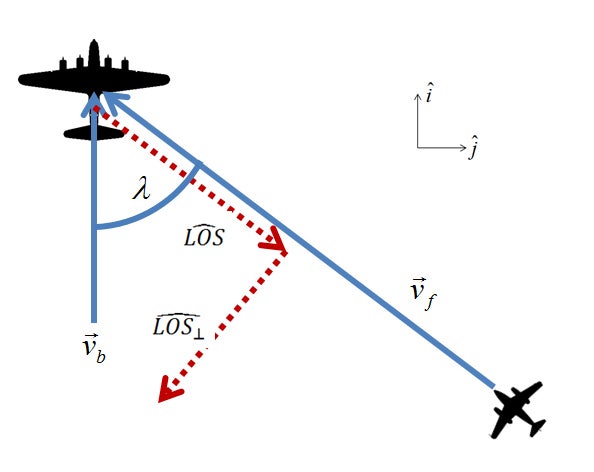
Given the diagram, the bomber and fighter velocity vectors (Vb and Vf respectively) can be written as,

The closing velocity vector (Vc) can then be written as,
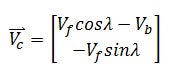
Lead is dependent only upon the Vc component along the unit vector orthogonal to the line-of-sight. The unit line-of-sight vector (LOS) and its orthogonal unit vector (LOS⊥) can be written as,
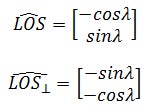
The dot product of Vc and LOS⊥ provides the apparent crossing velocity that the gunner must lead. This evaluates to,
![]()
It is interesting to note that only the bomber velocity contributes to lead. This corresponds to the qualification in the film that the “3, 2, 1” rule applies only “if you’re flying a straight course and traveling at a true airspeed of 225”. When λ is 90o, the bomber motion solely constitutes the apparent crossing velocity. At a tail chase condition, lead is not required as sinλ evaluates to 0. Lead is proportional to the apparent crossing velocity and further calculations do not contribute to the discussion at hand. The dot product is sufficient to illustrate the rule of thumb.
Trigonometry
The table below shows the influence of sinλ upon the “3, 2, 1” rule. For a starting point, it uses the 3 radii lead at 90o deflection given in the film.
| λ (deg) | sin(λ) | 3*sin(λ) |
| 90.00 | 1.00 | 3.00 |
| 45.00 | 0.71 | 2.12 |
| 22.50 | 0.38 | 1.15 |
| 11.25 | 0.20 | 0.59 |
The astute reader will note that the discussion thus far assumed a constant range, In a real attack, the attacking fighter closed the range as it rolled in from beam to aft, necessitating less lead by the gunner. This effect is somewhat offset by the rounding down in the rule of thumb. The decreased range also minimizes the effects of error in lead. Lastly, it is doubtful that this neglect has any meaningful impact in the freezing and adrenaline-fuelled combat conditions.
The same trigonometric relationship also governs the application of wind drift ballistic data. 90o crosswind requires full value, 42o (generalized to 45o) requires 2/3 value, and 30o requires half value.
Technological Advances
Early war bombers such as the B-17 and B-24 required each gunner to be an organic ballistic computer. The more advanced B-29 featured a fire control system that automatically corrected for lead, bullet drop, and parallax between the gunner station and the remote turrets. However, the range and line-of-sight rates estimation still required gunner inputs. Setting a known target wingspan provided the arc length. Sizing the reticle to touch the target wingtips furnished the angle. Small angles approximation and trigonometry estimated the range to target. Lastly, the gunner tracked the target to provide line-sight-rates to the computer. Fighters utilized similar technology with the MK II Gyro gunsight (K-14 with USAAF, and MK18 with USN).
The incorporation of radar into the fire control system, such as the A-1CM gunsight on the F-86** and AN/APG-14 radar on the B-29, afforded an automated ballistic solution. During Operation Linebacker II of the Vietnam War, computer aided B-52 tail guns claimed two MIGs.
A friend of the author studied numerical analysis under Dr. Jack W Hollingsworth at Rochester Institute of Technology. The Dr. served as navigator and manned the cheek guns in the B-17 from 1942 to 1945. After the war, Dr. Hollingsworth worked for General Electric on radar directing the bomber defensive armament. In his teaching career, Dr. Hollingsworth used the gunnery problem as a vehicle to demonstrate differential equations. When bomber jackets were en vogue on campus during the 1980s, the Dr. would reminisce about his time over the frozen skies of Europe. After a lifetime of contribution to humanity, Dr. Hollingsworth passed away in 2012. http://www.legacy.com/obituaries/dailygazette/obituary.aspx?n=jack-w-hollingsworth&pid=160174410

B-52 Tail Gun radar
Citation
*AAF Manual 200-1: “Fighter Gun Harmonization”. Washington, D.C.: Headquarters of the United States Army Air Forces. 30 January 1945. p. 37.
https://www.scribd.com/fullscreen/125300050
** “Radar Gunsight A Partial Lifting of the Security Veil from American Fighter Equipment.” Flight, 16 Jan. 1953, pp. 66–66.
https://www.flightglobal.com/pdfarchive/view/1953/1953%20-%200068.html
 Your Privacy Choices
Your Privacy Choices
