Since we know that gunshot wounds follow physical laws – Newtonian mechanics, specifically – we can use physical quantities to describe what happens to a bullet when it enters a fleshy target. In a previous post, we were introduced to three physical quantities: Force, work, and kinetic energy. To see how these apply to a gunshot, let’s use the example of a hollow point bullet as it impacts and penetrates 10% ballistic gelatin.

Ballistic gelatin is essentially a solution of pure collagen (which is the primary protein in connective tissue) in water, and we can think of ballistic gelatin as acting much like water at the high speeds of a bullet impact. Indeed, jacketed hollow point bullets designed to expand in gel and tissue expand very nicely when shot into water, too. So first, let’s consider the design of a modern hollow point projectile, the below sectioned example being monolithic for simplicity’s sake:

We can see that the cavity in the bullet is bell-shaped. When the projectile is shot into gelatin, the media outboard of the cavity flows around the bullet, just like it would with a standard full metal jacket projectile. However, the gelatin in the path of the cavity itself has nowhere to go; it can enter the cavity, but then it becomes trapped as the bullet’s momentum carries the projectile forward. This creates a great deal of hydraulic pressure inside the cavity, which in hollow point bullets is harnessed to cause expansion. The forces generated by the forward movement of the projectile against the fluid act on the walls of the cavity, which have already been weakened during the manufacturing process by deep score marks. If the forces are high enough, the bell breaks into petals, which are then forced open even further by the oncoming gelatin, creating a “mushroom” or “flower” shape.

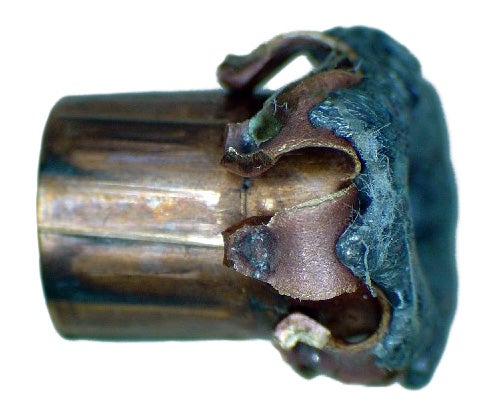
This expanded .38 Special hollow point bullet illustrates how the hydraulic pressure has forced the walls of the hollow cavity outward, rupturing them along pre-made fracture points that become the edges of petal-shaped structures.

These forces acting on the bullet and the tissues together comprise the work done by the projectile. This work is a function of the energy of the bullet as it loses velocity in the target. There is a misconception that it is the “energy dump” of a projectile that causes wounding, but that’s actually backwards. Since there is a relationship between energy and work, a very rapid energy dump and deceleration by a projectile implies a large amount of work being performed on the projectile and target in a very short amount of time. It’s this work, expressed by a deforming or tumbling projectile cutting or crushing tissue, that is the true actor in wounding.

This has some interesting implications if we also consider the structure of small arms projectiles. Let’s take a look at a very simple model of two fragmenting projectiles of the same design made of the same materials with a given toughness. First, remember that the physical property of toughness is a measure of how much energy a material can absorb per unit volume before it ruptures, given in units of Joules per cubic meter or foot-pounds per cubic foot. Toughness is far from the only mechanical property of a material that should be considered for a thorough analysis of projectile impacts, but to keep the example simple we will consider it alone.

Let’s consider a 55 gr (3.56 g) gilding metal jacketed lead projectile that is .224″ caliber. In order for this bullet to fragment, the gilding metal jacket must rupture under the force of impact. Due to its design and toughness, this can occur only at energies of 1,000 Joules or higher. We can calculate the minimum fragmentation velocity of the bullet by solving the kinetic energy equation for velocity:

1,000 J = 0.5 * 3.56 g * .001 kg/g * V^2

Which gives us 750 m/s velocity, or 2,460 ft/s as the velocity at which the projectile can fragment.

What if we double the weight of the projectile by increasing its size? Doing this gives us a projectile that is 0.282″ caliber and weighs 110 gr (7.13 g). Does this projectile still fragment at energies of 1,000 Joules or higher? If the projectile is scaled up exactly, the answer is “no”; the toughness of the projectile is given as per unit volume, which means that (assuming fixed density and the same construction), a jacket that is twice as heavy will require twice as much energy to rupture. Since the bullet is twice as heavy, we can see that the new equation solves out exactly the same as the old one:

2,000 J = 0.5 * 7.13 g * .001 kg/g * V^2

V = 749 m/s

This is one reason we observe that velocity is so important for terminal effectiveness. There are only two ways to increase the energy of a projectile: Increase the velocity, or increase the bullet weight. However, increasing the bullet weight often (though not always) also increases the durability of the bullet and raises the amount of energy needed to cause upset to the projectile in the form of expansion, fragmentation, or another effect. Therefore, it is by raising the velocity of a projectile that the most dramatic gains in wounding are often seen; this is simply a way to raise the projectile’s energy (and therefore ability to do work) without also making the projectile tougher and more resistant to deformation.

It is more difficult, but becoming increasingly common, to design projectiles that require less force (and thus energy and work) to deform or disrupt, and which therefore can do so at lower velocities than ever before. Good examples of rifle bullets that have been well-crafted to expand or fragment at much lower velocities than were previously normal include the new M855A1 and M80A1 EPRs, the Mk. 318 and Mk. 319 SOST projectiles, and mono-alloy bullets like those from Barnes. This solution, provided the resulting bullet is not too weak to hold up in flight, is an excellent one, and its widespread adoption has greatly increased the effectiveness of rifle calibers that previously suffered from poor performance.

This brings me to a core concept that I don’t think is often explicitly outlined. That is that higher impact energy allows for more work to be done, increasing the wounding potential of a projectile, but it does not ensure more severe wounding. In other words, kinetic energy (along with other less relevant quantities) is often treated in the literature as if it is a measure of the wounding power of a round. This is not the case; rather energy is more like a wounding budget, which can be spent very efficiently to produce more serious wounds, or very inefficiently, therefore producing lackluster wounds. This is how a .50 BMG round can produce less severe wounds than a 5.56mm round with a tenth of the energy, provided the former spends its energy inefficiently (e.g., by not upsetting and creating a “through and through” wound) and the latter spends its energy very efficiently (e.g., by fragmenting early and dramatically).

It seems useful, then, to coin the term energy budget as a shorthand for this concept. Using this term instead of “kinetic energy” helps to illustrate that the higher energy level may imply greater wounding capacity and terminal effectiveness, or it may not. This separates out the issue of empirically-observed terminal effectiveness, and makes it more clear why impact energy is a useful quantity to compare between rounds. For example, if I say that 7.62mm has more kinetic energy than 5.56mm, this implies to the listener/reader that I mean 7.62mm is a superior wounder, even if I don’t. However, saying 7.62mm has a higher energy budget than 5.56mm makes it clear that I mean there is more energy available in the larger caliber which can be used in the ways described in this series to cause more severe wounds, but not necessarily that 7.62mm will be the better wounder.

A great deal of research and development has been conducted to reach the level of understanding we have now, which has in turn given us some very effective projectiles even in modest calibers. Of course, it would be nice if there were a simple equation that would tell us exactly how effective a given round would be based just on its bullet weight, caliber, and velocity, but unfortunately the subject of wounding and lethality is far, far too complex for such simple models. Hopefully, though, what I’ve written here helps the understanding more than it hurts.